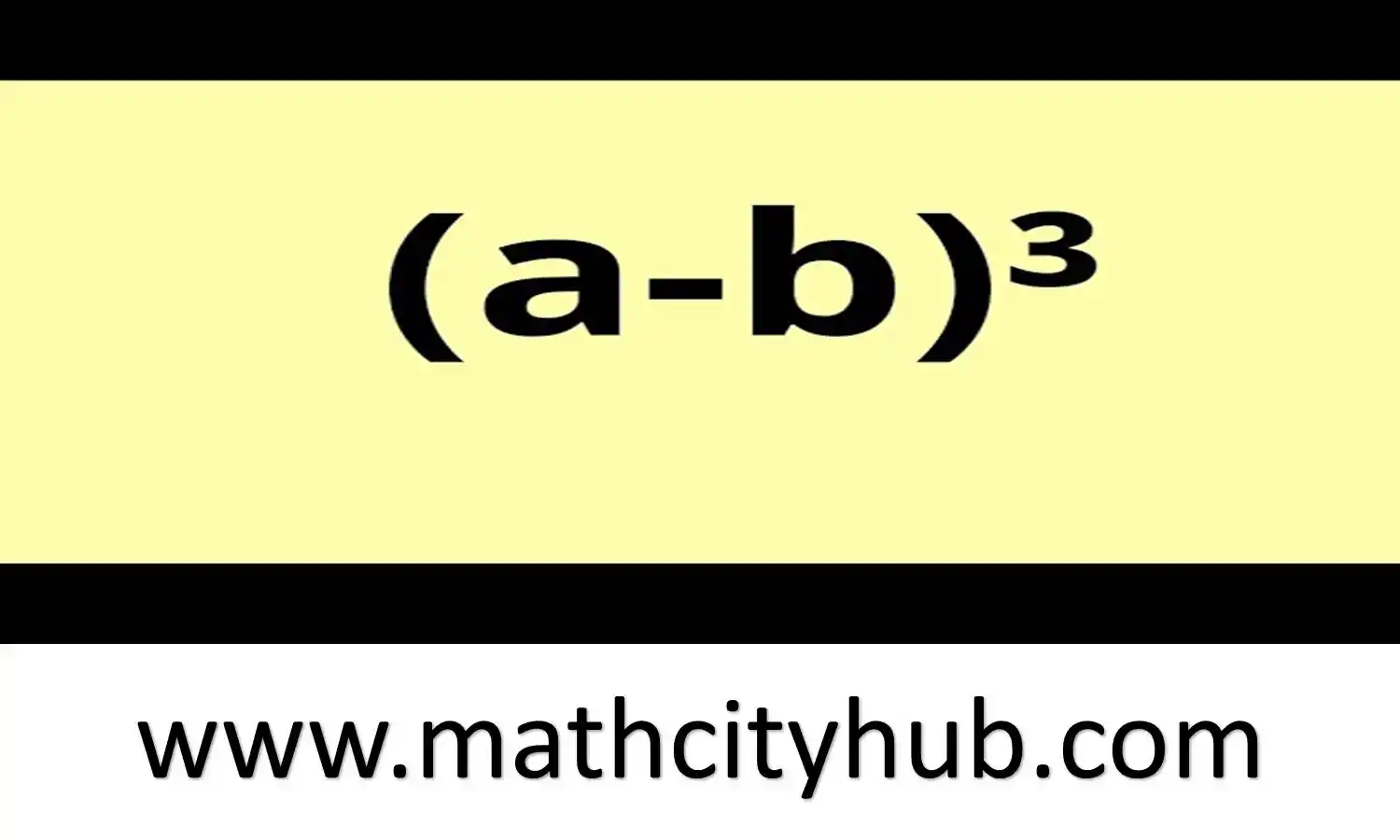
a-b Whole Cube – Let x and variables be in algebraic form, representing two mathematical terms. The distinction between them is expressed mathematically as x-y.
This is an algebraic expression. It is also the binomial. The Cube of distinction of two terms, or a binomial, can be described in the following form in mathematics : (x-y)3
The x minus the whole Cube equals the Cube of x divided by y by 3x times x, minus 3xy times x minus.
(x-y)3 = x3-y3-3xy(x-y)
This algebraic identity could be written using the following format too.
(x-y)3 = x3-y3-3x2y+3xy2
In general, the whole algebraic identity cubed is referred to in the following three methods in math.
The Cube of distinction between two terms or, more simply, the Cube of identity.
The Cube of the binomial formula. The binomial product special rule.
In reality, the Cube of distinction algebraic identity is utilized in two cases of special mathematics.
Expansion
The Cube of differences between two terms is enlarged by subtracting three times the average of the two terms and subtracting the third term from the first term, and subtracting a 2nd term’s Cube from that of the first term.
Simplification
a-b Whole Cube – Subtraction by three times the amount of product from both terms and that of subtracting the 2nd one from the first term and subtracting the first term’s Cube from the initial term. This is then referred to by naming the Cube of difference between the two terms.
The a-b whole Cube, i.e., (a-b) 3 formula, is used to determine the Cube of the differences between two different terms.
The formula can also be used to manufacture certain types of trinomials. The whole cube formula is among the most essential mathematical identities.
Typically, this (a-b) 3 formula can be used to solve the problem quickly and without complex calculations. In this post, we will explore the entire cube formula, its explanation, and examples in depth.
Also Read: A Plus B Whole Square & Cube
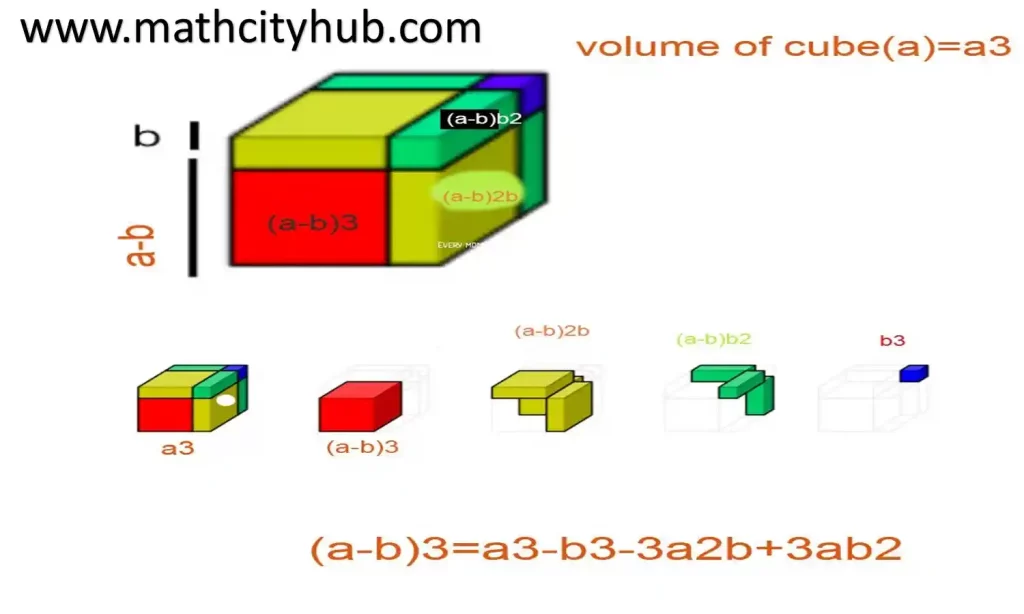
A-B Whole Cube Formula
(a-b) 3formula is used to determine the Cube of the binomial. (a-b) 3 is not a thing more than (a-b)(a-b)(a-b). The formula for the whole Cube is derived from the following:
(a – b)3 = a3 – 3a2b + 3ab2 – b3
(a-b)^3 Formula Derivation
To find from the equation for (a-b) 3, We must multiply (a-b) three times. (i.e.) (a-b)(a-b)(a-b). Read the following steps to discover how to calculate (a-b) 3.
Derivation:
- (a-b)3 = (a-b)(a-b)(a-b)
- (a-b)3 = (a2-2ab+b2) (a-b) [Since, (a-b)2 = a2+b2-2ab)
- (a-b)3 = a3-2a2b+ab2-a2b+2ab2-b3
- (a-b)3 = a3-3a2b+3ab2- b3
- The equation for (a-b)3 is a3-3a2b+3ab2-b3.
The formula above can be written in the form:
(a-b)3 = a3-3ab(a-b) – b3.
Also Read: Types of A Specific Formula for Solving a Problem Is Called
Also, check out:
Monomial & Polynomial
Algebraic Identities for Class 9
Examples on (a-b)^3
Example 1: Find the answer to the expression (x-2y) 3.
Solution:
- Given expression: (x-2y)3.
- We are aware that (a-b)3 is a3-3a2b+3ab2-b3
- In the equation (x-2y), 3 is x. A = 3 and 2y = b.
- Then, by substituting the value of the a-b entire cube formula, you receive
- (x-2y)3 = x3- 3(x)2(2y) + 3(x)(2y)2 – (2y)3
- (x-2y)3 = x3 – 6x2y+12xy2 – 8y3.
- Hence, (x-2y)3 = x3 – 6x2y+6xy2 – 8y3.
Example 2: Find the formula: (2x – 7y) 3
Solution:
- Given: (2x – 7y)3.
- We all know that (a-b)3 = a3-3a2b+3ab2-b3
- Here, a = 2x and b = 7y
- In the process of substituting values from an algebraic equation, we obtain
- (2x – 7y)3 = (2x)3 – 3(2x)2(7y) + 3(2x)(7y)2 – (7y)3
- (2x -7y)3 = 8×3 – 84x2y +294xy2 – 343y3
- Therefore, (2x – 7y)3 = 8×3 – 84x2y +294xy2 – 343y3.
The whole cube formula a-b, i.e., (a-b) 3 formula, is used to determine the Cube representing the difference between two different terms. This formula is also utilized to manufacture certain types of trinomials.
The a-b whole cube equation is one of the most significant mathematical identities. Typically it is the (a-b) 3 formula can be used to solve problems quickly without having to do any complex calculations.
In this post, we will look at the entire cube formula, details of its derivation, and even examples.
Also Read: Explanation Of A Square B With Example
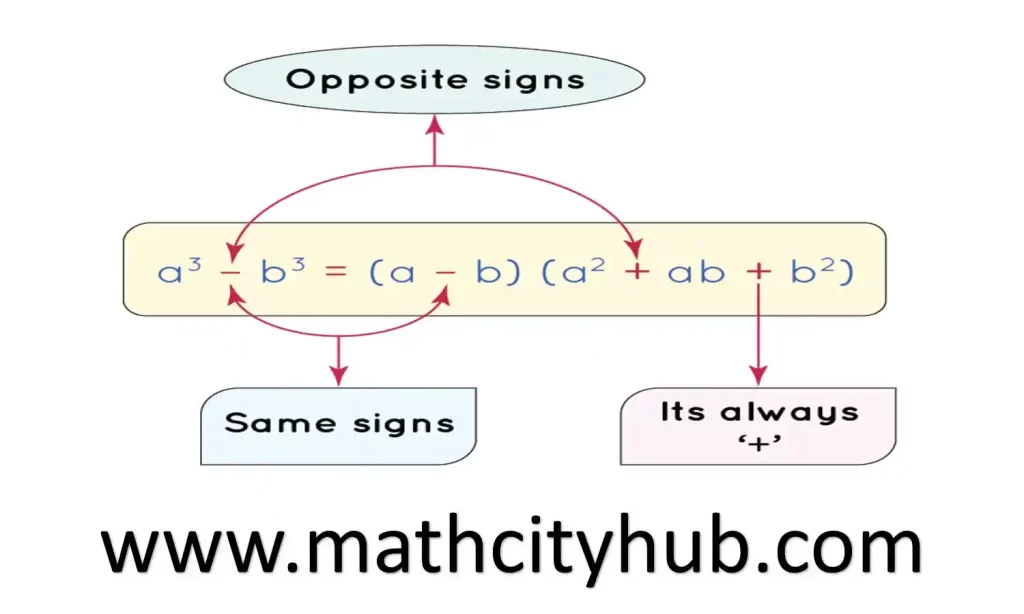
A MINUS B WHOLE CUBE FORMULA
Here, we’re going to examine the formula/expansion of
- (a – b)3
- That is,
- (a – b)3 = (a – b)(a – b)(a – b)
- Multiply (a – B) in addition to (a – B).
- (a – b)3 = (a2 – ab – ab + b2)(a – b)
- Simplify.
- (a – b)3 = (a2 – 2ab + b2)(a – b)
- (a – b)3 = a3 – a2b – 2a2b + 2ab2 + ab2 – b3
- Combining similar terms.
- (a – b)3 = a3 – 3a2b + 3ab2 – b3
- or
- (a – b)3 = a3 – b3 – 3ab(a – b)
Solved Problems
Problem 1 : Expand :
- (x – 1)3
- Solution :
- (x 1.) 3 is in the form of (a – (a -) 3
- When we compare (a – B) 3and (x 1) 3, we will get
- A = x
- B = 1
Write the formula or expansion for (a – 3.) 3.
- (a – b)3 = a3 – 3a2b + 3ab2 – b3
- Substitute x in place of a and 1 for.
- (x – 1)3 = x3 – 3(x2)(1) + 3(x)(12) – 13
- (x – 1)3 = x3 – 3×2 + 3(x)(1) – 1
- (x – 1)3 = x3 – 3×2 + 3x – 1
- Thus, the expansion of (x 1) 3 is
- x3 – 3×2 + 3x – 1
Problem 2 : Expand : (2x – 3)3
Solution :
- (2x (3) 3 is in the form of (a – (a -) 3
- When we compare (a – (b) 3 and (2x 3) 3, we will get
- A = 2x
- B = 3
- Create the formula or the expansion of (a – 3.) 3.
- (a – b)3 = a3 – 3a2b + 3ab2 – b3
- Substitute 2x for A and 3 for the letter b.
- (2x – 3)3 = (2x)3 – 3(2x)2(3) + 3(2x)(32) – 33
- (2x – 3)3 = 8×3 – 3(4×2)(3) + 3(2x)(9) – 27
- (2x – 3)3 = 8×3 – 36×2 + 54x – 27
- The increase to (2x -3) 3 is
- 8×3 – 36×2 + 54x – 27
Problem 3 : Expand :
- (x – 2y)3
- Solution :
- (x 2y) 3 is in the form of (a – (a -) 3
- When we compare (a – (a -) 3 and (x 2y) 3, we will get
- A = x
- B = 2y
- Create the formula/extension for (a – 3.) 3.
- (a – b)3 = a3 – 3a2b + 3ab2 – b3
- Substitute x for A and 2y for.
- (x – 2y)3 = x3 – 3(x2)(2y) + 3(x)(2y)2 – (2y)3
- (x – 2y)3 = x3 – 6x2y + 3(x)(4y2) – 8y3
- (x – 2y)3 = x3 – 6x2y + 12xy2 + 8y3
- The expansion of (x 2y) 3 is
- x3 – 6x2y + 12xy2 + 8y3
Problem 4 :
- If a – B = 3 and 3 + 3 – 3 = 1197, and a 3 – 3 = 1197, determine the value of ab.
- Solution :
- We can use the formula or expansion to calculate (a + (b) 3 to determine the value of ab.
- Write the formula or the expansion of (a – (a -) 3.
- (a – b)3 = a3 – 3a2b + 3ab2 – b3
- or
- (a – b)3 = a3 – b3 – 3ab(a – b)
- Substitute 13 for (a – B) and 1197 to substitute for (a 3 – 3). 3).
- (3)3 = 1197 – 3(ab)(13)
- Simplify.
- 27 = 1197 – 39ab
- Subtract 1197 on each side.
- -1170 = -39ab
- Divide each side in half (-39).
- 30 = ab
- The value of ab is 30.
Problem 5 : Find out the value of :
- (98)3
- Solution :
- We can apply the algebraic formula (a + B) 3 and easily find the value of (98) 3.
- Create (98) 3 as (a – 3.) 3.
- (98)3 = (100 – 2)3
- Make the necessary expansions for (a – B) 3.
- (a – b)3 = a3 – b3 – 3ab(a – b)
- Substitute 100 for A and 2 for the letter b.
- (100 – 2)3 = 1003 – 23 – 3(100)(2)(100 – 2)
- (100 – 2)3 = 1000000 – 8 – 3(100)(2)(98)
- (100 – 2)3 = 1000000 – 8 – 58800
- (98)3 = 941192
- Therefore, the amount in (107) 3 is
- 941,192
Also Read: Derivation of A Square Minus B Square With Example
A plus B Whole Cube
Are you in search of a minus-b whole Cube? It is possible to check the formulas for the whole Cube using three different ways. We will provide you with these (a-b)^3 algebra formulas, how to make (A-B)^3, and the proof.
We can write (a-b)3=(a-b)2x(a-b)
We know the formulas for a minus b complete square. a-b)2=a2+b2-2ab apply the formulas.
- =>(a-b)3=(a2+b2-2ab)x(a-b)
- =>(a-b)3=ax(a2+b2-2ab)-bx(a2+b2-2ab)
- Multiplication begins at the beginning
- =>(a-b)3=a3+ab2-2a2b-ba2-b3+2ab2
- Arrange
- =>(a-b)3=a3+ab2+2ab2-2a2b-ba2-b3
- =>(a-b)3=a3+3ab2-3a2b-b3
- Also Write : =>(a-b)3=a3-b3+3ab(b-a)
(A-B)^3 Verifications
Confirming whether the (a-b)3 formula is correct or incorrect is important. Enter the formula a=5 and b=3 . Place the values of a and B in the LHS
- =>(a-b)3=(5-3)3
- =>(a-b)3=(2)3
- =>(a-b)3=8
Put the value of a and B in the RHS
- =>a3+3ab2-3a2b-b3
- =>53+3x5x32-3x52x3-33
- put the value of square and Cube =>125+3x5x9-3x25x3-27
- Start multiplication = 125+15×9-75×3-27
- =>125+135-225-27
- Addition = 260-252
- Simplify Calculation 8
- Therefore LHS=RHS
Sure! Here are a few instances of expanding the binomial cube formula (a + b)3:
- Example 1 Expanding (a + b)3 by using the binomial Theorem:
- (a – b)3 = a3 – 3a2b + 3ab2 – b3
- Example 2: Extending (2a 3b)2 to (2a 3b)3 using the binomial Theorem:
- (2a – 3b)3 = (2a)3 – 3(2a)2(3b) + 3(2a)(3b)2 – (3b)3 = 8a3 – 36a2b + 54ab2 – 27b3
- Example 3: Extending (x + y)3 by using the binomial Theorem:
- (x – y)3 = x3 – 3x2y + 3xy2 – y3
- Example 4 Expansion (4x + 5y)3 by using the binomial Theorem:
- (4x + 5y)3 = (4x)3 + 3(4x)2(5y) + 3(4x)(5y)2 + (5y)3 = 64×3 + 240x2y + 300xy2 + 125y3
- These examples illustrate the use of the binomial theory to extend the Cube of the binomial expression (a – b)3 where both a and B represent variables or constants.
- Proof Formulas (a-b)3=a3+3ab2-3a2b-b3
- Summary (A+B)^3
If you need any help regarding the (A-B)^3 formulas, please let me know via email or social media. A + B Whole Cube is the most critical algebra math formula for the class of 6-13.
Also Read: Best 7th Grade Math Formulas for Children
Questions & Answers Derivation Of a-b Whole Cube
Here are some questions and answers on the derivation of the (a-b)3 formula:
Question 1: What is the (a-b)3 formula?
Answer: The (a-b)3 formula is an algebraic identity that states that the cube of the difference of two terms is equal to:
(a-b)3 = a3 - 3a2b + 3ab2 - b3
Question 2: How do you derive the (a-b)3 formula?
Answer: The (a-b)3 formula can be derived by expanding the product (a-b)(a-b)(a-b). This can be done using the distributive property of multiplication:
(a-b)(a-b)(a-b) =
(a)(a-b)(a-b) - (b)(a-b)(a-b)
The first term in the expanded product can be simplified using the square of a difference pattern:
(a)(a-b)(a-b) = a2 - ab(a-b)
The second term in the expanded product can also be simplified using the square of a difference pattern:
(b)(a-b)(a-b) = b2 - ab(a-b)
Combining the simplified terms, we get the (a-b)3 formula:
(a-b)3 = a2 - ab(a-b) - ab(a-b) - b2
Question 3: What are some examples of how the (a-b)3 formula can be used?
Answer: The (a-b)3 formula can be used to solve problems involving the cube of the difference of two terms. For example, if we want to find the cube of 5-2, we can use the (a-b)3 formula to get:
(5-2)3 = 53 - 3(5)(2) + 3(5)(2) - 23
Simplifying the expression, we get 125-30+30-8 = 97.
The (a-b)3 formula can also be used to factorize some types of trinomials. For example, the trinomial x3-6×2+9 can be factored as (x-3)(x2+3x-3) using the (a-b)3 formula.
I hope this helps! Let me know if you have any other questions.
Also Read: Easy Way to Learn A Cubed minus B Cubed
Conclusion:
a-b Whole Cube – The conclusion of the derivation of the (a-b)3 formula is that the cube of the difference of two terms is equal to:
(a-b)3 = a3 - 3a2b + 3ab2 - b3
This formula can be used to solve problems involving the cube of the difference of two terms, and it can also be used to factorize some types of trinomials.
Here is a summary of the steps involved in the derivation of the (a-b)3 formula:
- Expand the product (a-b)(a-b)(a-b) using the distributive property of multiplication.
- Simplify the first term in the expanded product using the square of a difference pattern.
- Simplify the second term in the expanded product using the square of a difference pattern.
- Combine the simplified terms to get the (a-b)3 formula.